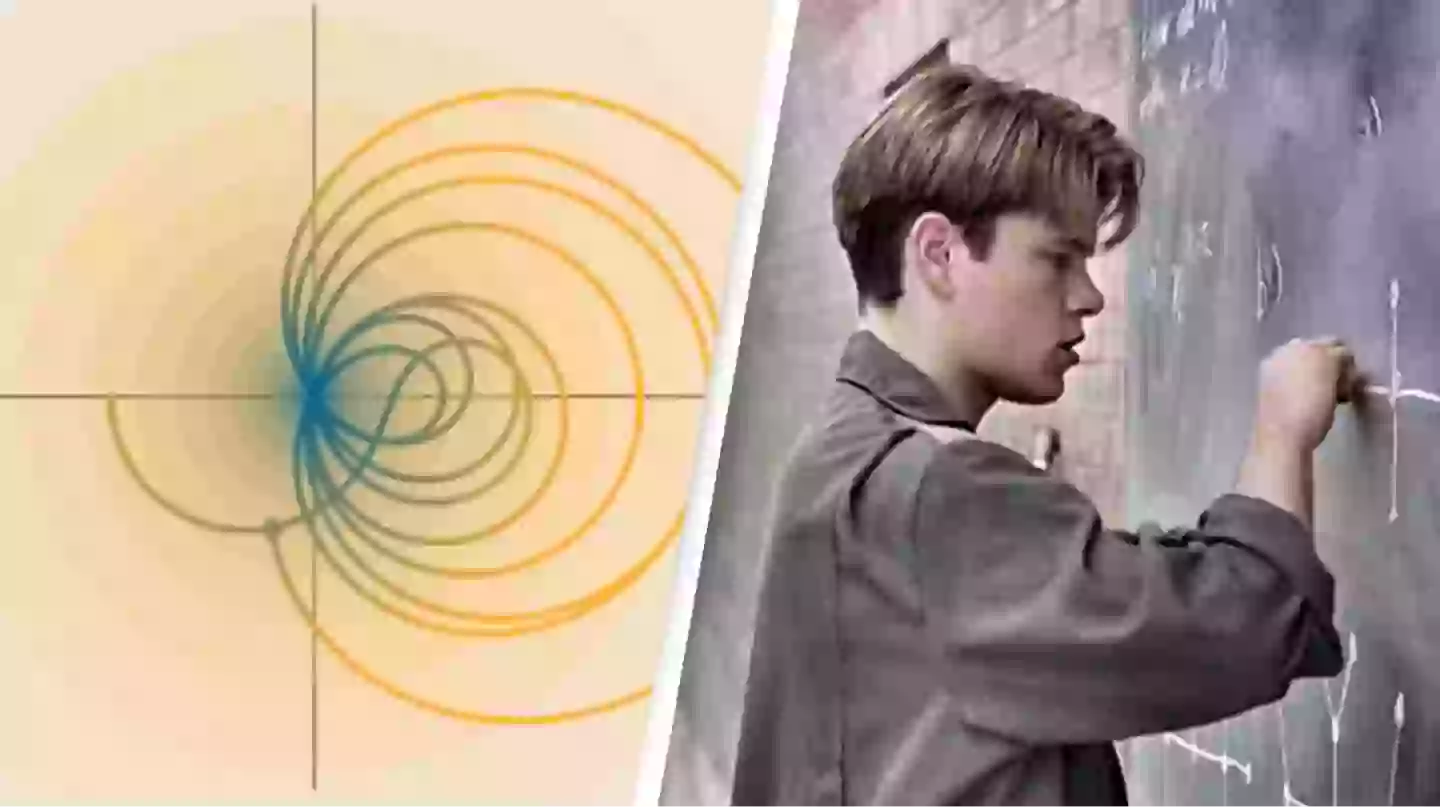
There’s a math question called the Riemann Hypothesis which can earn you $1 million for solving it.
The Riemann hypothesis was first proposed by German mathematician Bernhard Riemann in a 1859 paper ‘Ueber die Anzahl der Primzahlen unter einer gegebenen Grösse’ (‘On the Number of Prime Numbers Less Than a Given Quantity’).
Put in the simplest terms, it’s all about the distribution of prime numbers.
Or, as the Clay Mathematics Institute more eloquently puts it: “The prime number theorem determines the average distribution of the primes. The Riemann hypothesis tells us about the deviation from the average.
Advert
"Formulated in Riemann’s 1859 paper, it asserts that all the ‘non-obvious’ zeros of the zeta function are complex numbers with real part 1/2.”
If solved, it would have huge implications in number theory and encryption, meaning it is sometimes referred to as the ‘Holy Grail of Mathematics’.
Of course, it might seem natural to zone out slightly when talking about prime numbers and encryption, but what if we told you that solving the notoriously tricky hypothesis lands you a whopping $1 million?

Advert
Back in 2000, the mathematical conjecture was designated as a Millennium Problem, one of seven mathematical quandaries selected by the Clay Mathematics Institute of Cambridge, Massachusetts, which says of the Riemann Hypothesis: “This has been checked for the first 10,000,000,000,000 solutions.
"A proof that it is true for every interesting solution would shed light on many of the mysteries surrounding the distribution of prime numbers."
The solution of any of these seven Millennium Problems will land you a cool $1m, so it might be worth getting your calculator out.
Mathemetician, comedian and former teacher Matt Parker told the Guardian back in 2010 that experts are ‘obsessed with primes because they are the foundation of all other numbers’.
Advert
He said: “Prime numbers in mathematics are like atoms in chemistry, bricks in the construction industry and ludicrous pay cheques in professional football.
“Everything is built up from these fundamental units and you can investigate the integrity of something by taking a close look at the units from which it is made.

“To investigate how a number behaves you look at its prime factors, for example 63 is 3 x 3 x 7. Primes do not have factors: they are as simple as numbers get.”
Advert
He also tipped others off with an ‘easier starting problem’ for solving the Riemann hypothesis: “All prime numbers (greater than five) squared are one more than a multiple of 24.
"Check it for a few – it works. You can even prove that it works for all of the infinite number of primes.
“Now if you can just do that for the Zeta zeroes, you can stop kicking a football around in the cold in hope of a big pay day.”
Good luck...
Topics: World News